Polymer Physics
Michael Lang, Jens-Uwe Sommer, Martin Wengenmayr and Ron Dockhorn
Computer simulations offer a unique possibility to develop theoretical models for complex soft matter or polymer model systems. When starting from the same assumptions as theory, one can test numerically the predictions of theoretical models. Comparison with experimental data provides additional insight into the differences between real samples and the assumptions made for simulations or theory. A combination of both strategies helps to bridge the gap between theory and experiment to finally develop accurate models for complex matter. Or it allows to solve pending problems of polymer physics that have been proposed but not been sorted out yet.
Similar strategies have been used in recent years to address special physics problems related to the interplay between topology and conformations of polymers, the properties of polymer melts, thin films, or grafted polymers. Future work in this field may cover polymer model systems with nano-engines, dynamics in confined environments, or other unsolved problems that require additional input from simulations.
Condensation reactions lead typically to a broad molecular weight distribution, in particular in the case of a co-polycondensation, where high molecular weight species are only obtained for stoichiometric mixtures of the two reacting monomers. By using a third species that competes with one of the original species for reactions, one can make use of fact that irreversible reactions can replace exchange reactions [1]. This introduces two peaks for the weight average molar mass where either the dominant competitor is in a stoichiometric ratio with the alternating partner or the sum of both competitors is in a stoichiometric ratio with the partner. The interesting point is that the position of the second peak can be tuned by the amount of the subdominant competitor. In between both peaks, a zone with stable reaction conditions (essentially independent of small composition fluctuations) arises, which can be used to minimize the polydispersity of the resulting co-polymer.
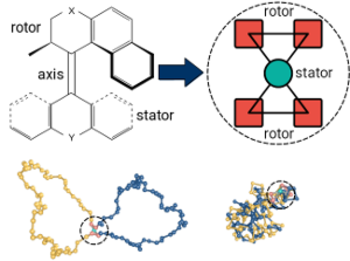
The physics of knotting is a complex problem that is highly relevant to understand the properties of giant molecules such as DNA. To date, there is still no agreement on basic properties of knotting as, for instance, on the localization of a knot on a self-avoiding walk. This stems from the fact that the simulated molecules may have been to small to accommodate all of the knot in an unbiased way along their contour. We circumvented this problem by the utilization of a Feringa nano-engine that allows to continuously increase the self-entanglements of finite polymers from zero up to a fully entangled state [2]. The Figure shows on top left the chemical structure of this molecular engine that is coarse grained in our Monte-Carlo simulations to the structure on the top right. Feringa engines convert the energy of light into rotational motion, which coils up two cyclic strands against each other as shown in the bottom of the Figure. This set-up allows to measure the entropic penalty for creating extra confinement blobs within the coiled part of the molecule and localization can be analyzed from the increase of the winding number as a function of applied torque at the engine. Our results are in line with weakly localized knots on a-thermal polymers and provide a strong support for previously published physics models on the conformations of knotted polymers.
One rather surprising observation of several simulation studies was that the relaxation behavior of grafted chains may still be governed by Rouse dynamics, even though the overlap number of the strands was well in the entangled regime, where the dynamics of a corresponding solution of star polymers were dominated already by retraction dynamics. We could show that the classical analysis of the end-to-end vector auto-correlation function is not suitable for analyzing retraction dynamics [3]. With a modified analysis we demonstrated that the relaxation of densely grafted layers of large polymers is indeed dominated by arm retraction and requires a time ~ N3exp(N) as a with a function of the degree of polymerization N that was predicted theoretically.
One particular puzzling point of polymer physics was for decades that the Flory theorem could not be verified by experimental data [4]. This problem is of fundamental interest as it challenges the concept of excluded volume that is the text book basis for modeling real polymers. This problem could be solved by considering additional corrections due to bond-bond correlations, a contribution of chain ends, and the overlap limit of the chains. With these corrections, the data follows the originally proposed cross-over from mono-disperse melt to a-thermal solutions [4].
A brief discussion of somewhat less recent results is available here.
Publications
- Lang, M. ; Böhme, F.
Competing exchange and irreversible reactions in a linear co-polycondensation lead to a broad composition window where tuneable high molecular weight polymers can be produced
Macromolecular Theory and Simulations 28 (2019) 1800069. - Lang, M. ; Schuster, C. ; Dockhorn, R. ; Wengenmayr, M. ; Sommer, J.-U.
Testing the physics of knots with a Feringa nanoengine
Physical Review E 98 (2018) 052501. - Lang, M. ; Werner, M. ; Dockhorn, R. ; Kreer, T.
Arm retraction dynamics in dense polymer brushes
Macromolecules 49 (2016) 5190-5201. - Lang, M. ; Rubinstein, M. ; Sommer, J.-U.
Conformations of a long polymer in a melt of shorter chains: generalizations of the Flory Theorem
ACS Macro Letters 4 (2015) 177-181.

